π Student Questions and Answers
New In-Section Whiteboard: robmunger.com/2700whiteboards
(The above link will only be used during section; my main resources will still be here on this website.)
Click here to learn about timestamps and my process for answering questions. Section agendas can be found here. Email office hour questions to robmgmte2700@gmail.com. PS1Q2=βQuestion 2 of Problem Set 1β
π Questions covered Saturday, March 23
No questions covered.
π Questions covered Monday, March 25
Effective cost of debt
π£ 7:42
β When calculating the βeffective cost of debtβ , is the YTM or the coupon rate applied?
β For all costs of capital, you use the return that investors expect. YTM is the return of holding a bond. Therefore, the pre-tax cost of capital for bonds will be the YTM.
Coupon rates have nothing to do with the YTM, except in one special case. Bonds are often initially sold at their face value (in the primary market - when they are sold by the firm to investors for the first time). When a bond is sold at itβs face value (we also sometimes say, βat parβ - keep an eye out for this) then the YTM is equal to its face coupon rate.
Taxes and cost of debt
π£ 8:20
β Hi Rob, could you please elaborate a little more on the taxes and the cost of debt slide and a visual example of this impact in the income statement?
β The pretax cost of debt is the return on debt, $r_d$, which is measured as the Yield to Maturity. After-tax or βeffectiveβ cost of debt is this times (1-Tc), where Tc is the corporate tax rate. Multiplying by (1-Tc) lowers the cost of debt. In other words, it is relatively cheap to raise financial capital by issuing debt. This explains why so many companies like having high debt loads.
Why does this work? Well, suppose that your debt investors require a 8% return and have a 25% corporate tax rate. This says that your cost of raising debt financing is only 8%Γ(1-25%)=6%. This means that you can pay your investors an 8% interest rate (or yield) on their bonds. However, if you find a project that has an IRR of 6.1%, you can still take that project on profitably. The reason that this works is that you are getting a return on the project of 6.1%, but you are also getting a tax shield from the debt. The tax shield is worth 8%Γ25%=2%. Therefore, your total benefits arenβt just 6.1%, they are 6.1%+2%=8.1%, and 8.1% is more than your pretax cost of debt financing. There is a positive .1% spread that you are making, so you are increasing shareholder value.
Takeaway: multiply by (1-Tc) almost all of the time. It captures the benefit of the tax shield given by paying interest. Ask me more questions!
Slide 8
π£ 8:41
β S8, r(wacc) formula, please give example.
β This WACC formula is the same formulas that is on the following slide:
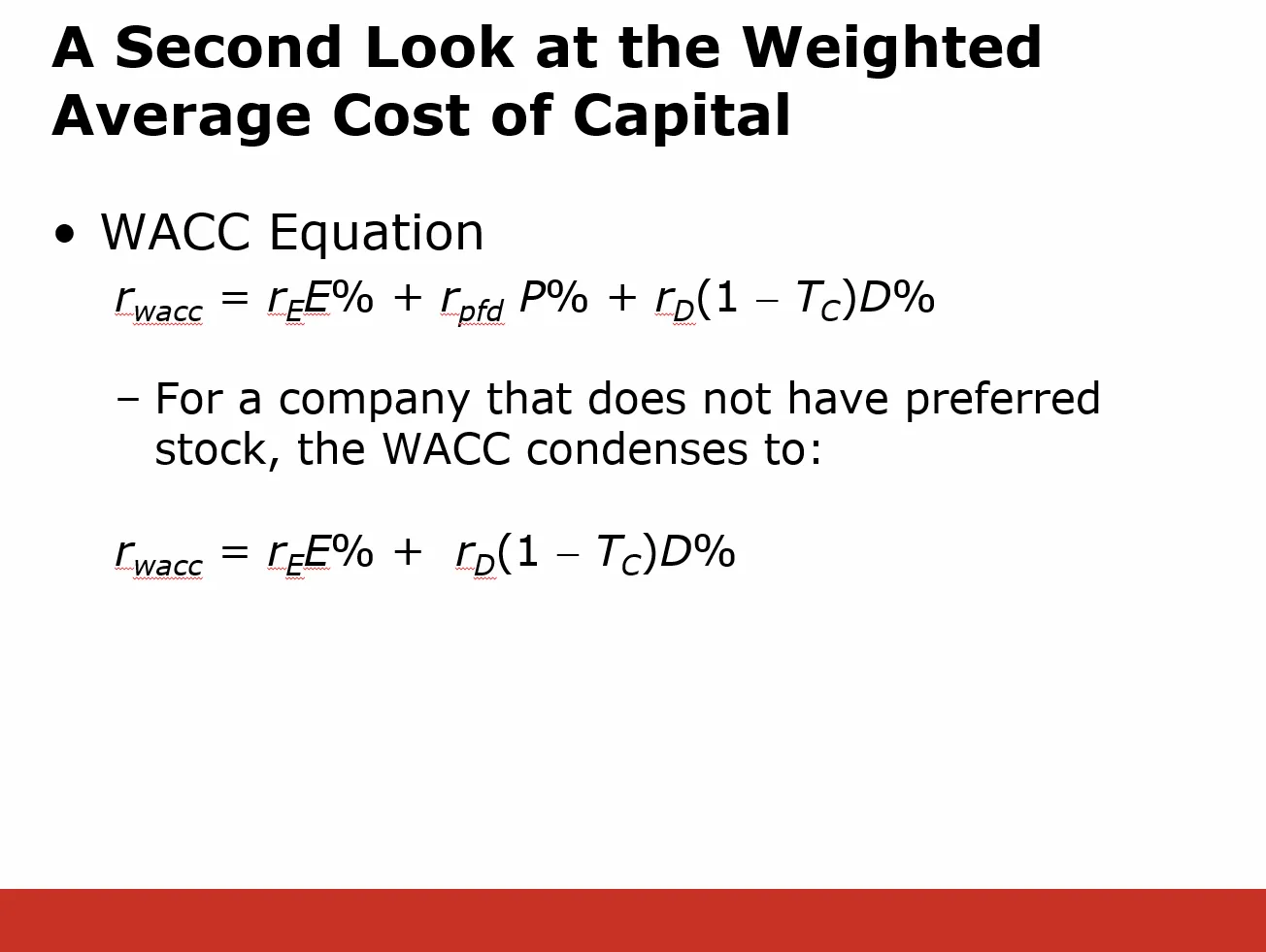
Itβs the same formula we used elsewhere during sections. See the page on Understanding WACC for details. We reviewed it at the start of todayβs section.
Slide 34
π£ 8:45
β S34: alpha, beta and CAPM. Please remind us again when we use formula without the Jensenβs alpha and which case we should use Jensenβs alpha.
β Youβll almost never use Jensenβs alpha. Itβs just there to help you understand what people mean when they say that a given company has alpha. It just means that the average return going forward is better than you would expect based on Ξ². The core WACC calculations assume Ξ±=0.
Slide 36
π£ 8:48
β S36: diagram.
How to find the market value of debt when debt has both coupon rate and yield to maturity rate. The investors want to compensate for the risk, using the yield to maturity per Bruceβs slides. Please give an example of calculation.
β Covered earlier. See the question above about coupon rate. Coupon rate can be ignored unless it helps you calculate out YTM. As mentioned above if a bond is selling at par (ie at face value), then its coupon rate = itβs YTM = itβs pre-tax cost of debt
PS5Q4
π£ 8:50
β To determine the expected return on assets are we just taking the new asset value minus the old asset value and dividing by the original asset value? [YES] If so, I have my answer for the assets going up versus the assets going down. To determine the expected return on assets, I assume I am assigning a 50% weight to each since it says the outcomes are equally likely [YES]. Once I figure out this, and it asks me the expected return on debt, I am multiplying the expected return on assets by the debt weight.
Iβm not understanding the remaining part of the problem and unsure how to ask a specific question regarding it. Any way you can point me in the right direction with the remaining parts of the problem?
β We covered this question in great depth on Saturday. In general, for any debt, if you are confident that the debt will be repayed, then the expected return on the debt equals either the interest rate (if a loan) or the YTM (if a bond). Mostly, though, see the middle portion of the Saturday recording.
PS5Q5
π£ 7:51, 8:37
β What does it mean by expected excess return of the market portfolio? Is this the market premium?
β Expected Excess return of the market portfolio is a synonym for Expected Risk premium of the market/ on the market. SEE ALSO THE QUESTION BELOW.
Market Risk Premium (Slides 46-47)
π£ 8:37
βWhy, on slide 46-47, does he just plug in the market risk premium instead subtracting off the risk free rate.
β This is because the market risk premium is different from the expected return on the market. In fact,
You donβt have to subtract off rF because itβs already been subtracted for you.
Premium means βextra,β so a risk premium is the extra return you get beyond the risk-free rate:
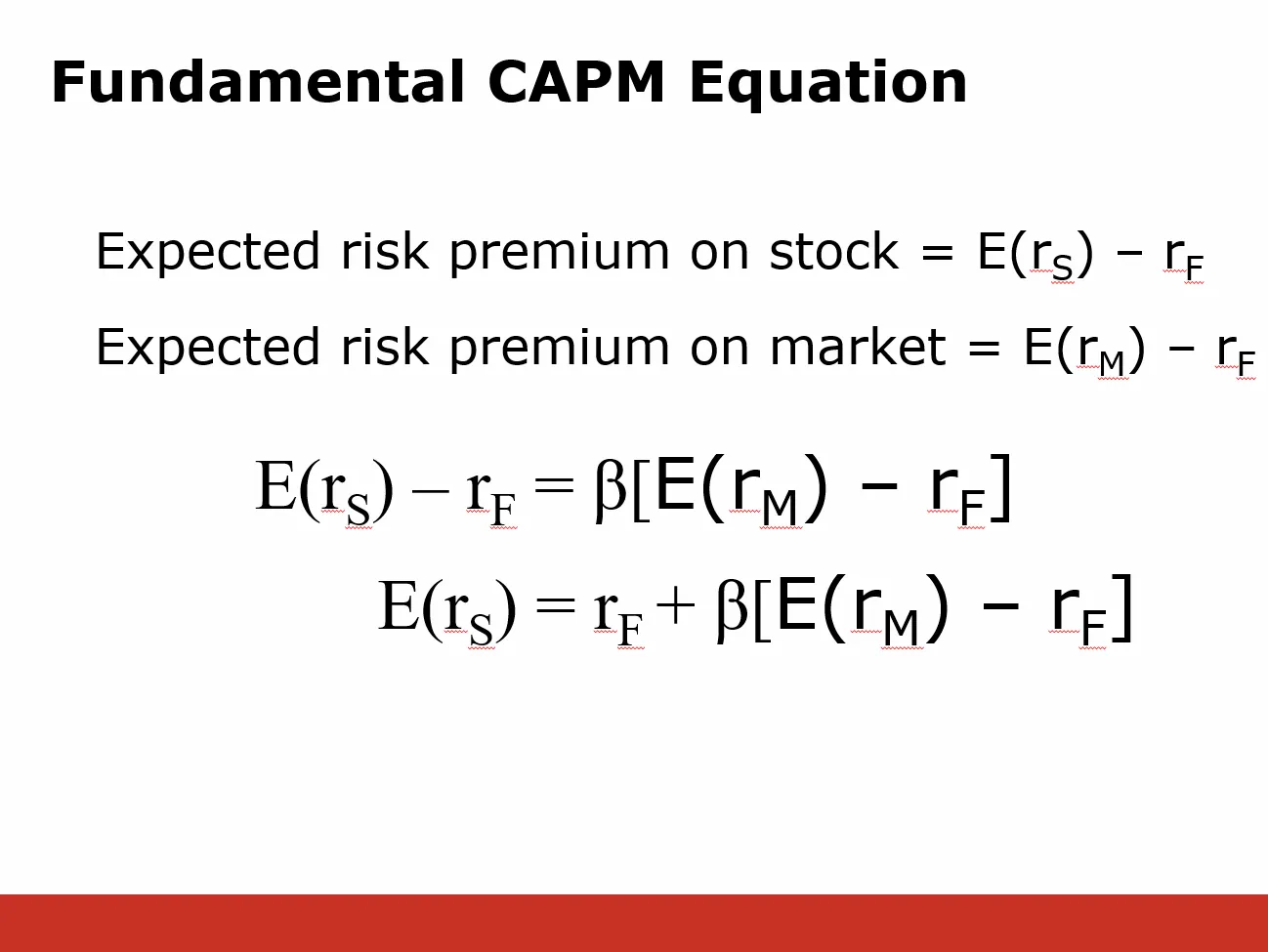
PS5Q4
π£ 8:50
Q4: expected return on the portfolio pre tax: Because the debt stays the same, the 40% debt and 60% equity on the portfolio is slightly changing when we are using the overall expected return of the firm/ assets. So, which weight should we use to calc pretax WACC. The orginail 40%D :60%E or the slight change 39%D :41%E? Please help. Thanks Rob.
β use the initial weights - ie 40%-60%.
PS5Q5
π£ 9:07
Q5: the result 7.75% equals 7.75 percentage points. Is this correct interpretation?
β See video. If one number is 7.00% and the other is 7.75%, then the difference is 0.75 percentage points.
Risk premium vs. expected return
π£ 9:11
In the Watson company WACC Example on the whiteboard it states that βthe return on T-Bills is 5% and the average return on the market, historically, is 15% note: the average return on the market can be used as your market risk premium/expected excess return on the market. β Would the 15% here be the βrisk premiumβ or the overall βMarket expected returnβ?
β Expected Return = Expected Return of Market = Expected Return on Market = Expected Return on Market Portfolio = 15%.
Market Risk Premium = Risk Premium of the Market = Risk Premium of Market Portfolio = 15%-5%=10%
Expected Risk Premium = Market Risk Premium = 10%.
How you remember this: Premium means βextra.β It is the return above and beyond the 5% risk free rate. A 15% expected return is only a premium of 10% over the risk free rate.